Here we answer many questions about the sequence -41, -48, -55, -62... What type of sequence is -41, -48, -55, -62? What is the next number in the sequence -41, -48, -55, -62? What is the nth number in the sequence -41, -48, -55, -62? What is the sum of the first 20 terms in the sequence -41, -48, -55, -62? What is the sum of the first n numbers in the sequence -41, -48, -55, -62?
In addition, we will also give you the formula that is used to calculate the next number or the nth number in -41, -48, -55, -62, and the formula to calculate the sum of n numbers in -41, -48, -55, -62.
A sequence is a list of numbers in a pattern, and each number in the sequence is called a term. We will use "terms" and "numbers" interchangeably on this page.
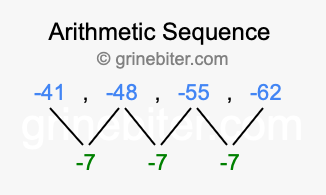
So, what type of sequence is -41, -48, -55, -62? The sequence -41, -48, -55, -62 has a common difference of -7 between each term. We call this kind of sequence an arithmetic sequence. Below is an image illustrating the correlation between the arithmetic sequence -41, -48, -55, -62 and its common difference of -7.
Now, what is the next number in the sequence -41, -48, -55, -62? Below is the formula used to calculate the next number in an arithmetic sequence, such as -41, -48, -55, -62. The first term listed in the sequence is "a", the common difference is "d", and "n" is the nth term of the arithmetic sequence.
a + (n-1) × d = Next Term
As stated above, the common difference (d) between each term is -7. Furthermore, the next term in -41, -48, -55, -62 is the fifth term (5), and the first term is -41. When we enter these values into our formula, we get the following answer:
-41 + (5 - 1) × -7 = -69
Thus, the next number (term) in the sequence -41, -48, -55, -62 is -69. The tool below calculates the nth term of the sequence using the formula above. For example, type in 20 if you want the 20th term in the sequence -41, -48, -55, -62, or 100 if you want the 100th term in the sequence -41, -48, -55, -62.
Let's move on to our next question. What is the sum of the first 20 terms in the sequence -41, -48, -55, -62? We use the formula below to calculate the sum of the first n terms in an arithmetic sequence such as -41, -48, -55, -62. Again, note that the first term is "a", the common difference is "d", and "n" is the nth term of the arithmetic sequence.
(n/2)((2 × a) + (n - 1) × d) = Sum
When we enter the a, d, and n values into our formula, where n is equal to 20, we can calculate the sum of all numbers up through the 20th term in the sequence -41, -48, -55, -62, as seen below:
(20/2)((2 × -41) + (20 - 1) × -7) = -2150
Therefore, the sum of all numbers up through the 20th term in the sequence -41, -48, -55, -62 is -2150. Below is another tool we created to make these calculations easier for you. This tool can calculate the sum of any number of terms in the sequence -41, -48, -55, -62 using the formula mentioned above. For example, if you type in 50, then it will calculate the sum of the first 50 terms in the sequence -41, -48, -55, -62.
Arithmetic Sequence Calculator
Go here to learn more about arithmetic sequences using the best online Arithmetic Sequence Calculator.
Sequence -40, -47, -54, -61
Here is the next sequence in our database that we have researched, defined, and explained for you.
Copyright | Privacy Policy | Disclaimer | Contact