Here we answer many questions about the sequence 35, 31, 27, 23... What type of sequence is 35, 31, 27, 23? What is the next number in the sequence 35, 31, 27, 23? What is the nth number in the sequence 35, 31, 27, 23? What is the sum of the first 20 terms in the sequence 35, 31, 27, 23? What is the sum of the first n numbers in the sequence 35, 31, 27, 23?
In addition, we will also give you the formula that is used to calculate the next number or the nth number in 35, 31, 27, 23, and the formula to calculate the sum of n numbers in 35, 31, 27, 23.
A sequence is a list of numbers in a pattern, and each number in the sequence is called a term. We will use "terms" and "numbers" interchangeably on this page.
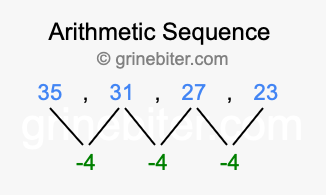
So, what type of sequence is 35, 31, 27, 23? The sequence 35, 31, 27, 23 has a common difference of -4 between each term. We call this kind of sequence an arithmetic sequence. Below is an image illustrating the correlation between the arithmetic sequence 35, 31, 27, 23 and its common difference of -4.
Now, what is the next number in the sequence 35, 31, 27, 23? Below is the formula used to calculate the next number in an arithmetic sequence, such as 35, 31, 27, 23. The first term listed in the sequence is "a", the common difference is "d", and "n" is the nth term of the arithmetic sequence.
a + (n-1) × d = Next Term
As stated above, the common difference (d) between each term is -4. Furthermore, the next term in 35, 31, 27, 23 is the fifth term (5), and the first term is 35. When we enter these values into our formula, we get the following answer:
35 + (5 - 1) × -4 = 19
Thus, the next number (term) in the sequence 35, 31, 27, 23 is 19. The tool below calculates the nth term of the sequence using the formula above. For example, type in 20 if you want the 20th term in the sequence 35, 31, 27, 23, or 100 if you want the 100th term in the sequence 35, 31, 27, 23.
Let's move on to our next question. What is the sum of the first 20 terms in the sequence 35, 31, 27, 23? We use the formula below to calculate the sum of the first n terms in an arithmetic sequence such as 35, 31, 27, 23. Again, note that the first term is "a", the common difference is "d", and "n" is the nth term of the arithmetic sequence.
(n/2)((2 × a) + (n - 1) × d) = Sum
When we enter the a, d, and n values into our formula, where n is equal to 20, we can calculate the sum of all numbers up through the 20th term in the sequence 35, 31, 27, 23, as seen below:
(20/2)((2 × 35) + (20 - 1) × -4) = -60
Therefore, the sum of all numbers up through the 20th term in the sequence 35, 31, 27, 23 is -60. Below is another tool we created to make these calculations easier for you. This tool can calculate the sum of any number of terms in the sequence 35, 31, 27, 23 using the formula mentioned above. For example, if you type in 50, then it will calculate the sum of the first 50 terms in the sequence 35, 31, 27, 23.
Arithmetic Sequence Calculator
Go here to learn more about arithmetic sequences using the best online Arithmetic Sequence Calculator.
Sequence 36, 32, 28, 24
Here is the next sequence in our database that we have researched, defined, and explained for you.
Copyright | Privacy Policy | Disclaimer | Contact